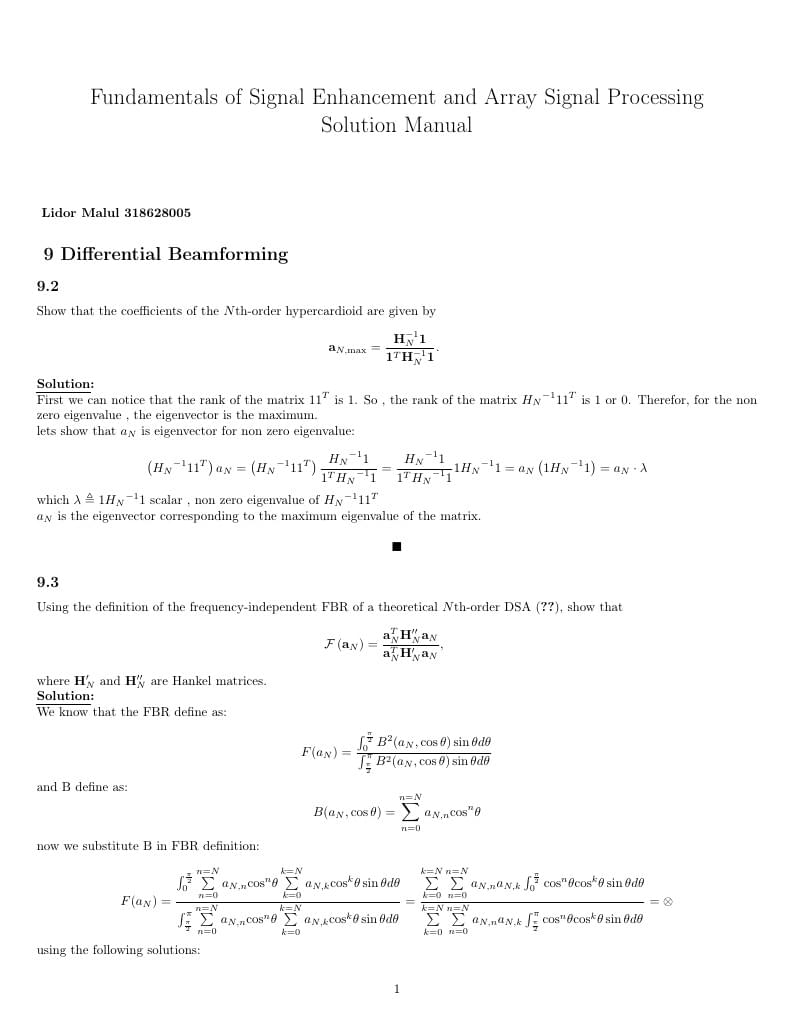
Community articles — Math
Seneste

This paper describes the three conditions of damped oscillations and the mathematical formulations of each condition.

Discrete maths for students of mathfac hse 19-23
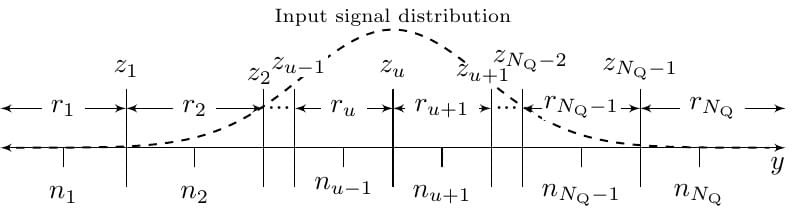
Hypothetical uniform quantizer standalone class figure.

Brief description of Gaussian Integral

Spring-Mass

section 10
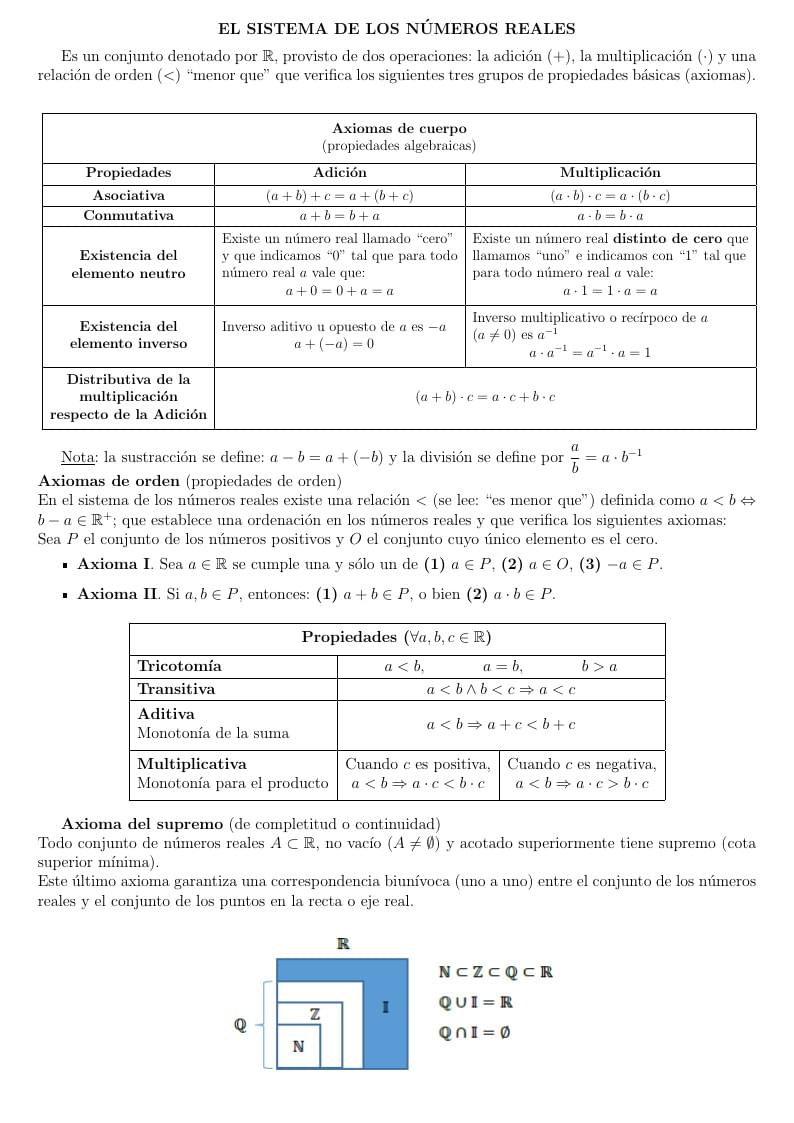
El sistema axiomático de los números reales. Los números reales forman un conjunto denotado por ℝ, provisto de dos operaciones: la adición (+), la multiplicación (⋅) y una relación de orden (<) “menor que” que verifica los siguientes tres grupos de propiedades básicas (axiomas).
\begin
Discover why over 20 million people worldwide trust Overleaf with their work.